Nuclear magnetic resonance (NMR) is a physical phenomenon in which nuclei in a magnetic field absorb and re-emit electromagnetic radiation. This energy is at a specific resonance frequency which depends on the strength of the magnetic field and the magnetic properties of the isotope of the atoms; in practical applications, the frequency is similar to VHF and UHF television broadcasts (60–1000 MHz). NMR allows the observation of specific quantum mechanical magnetic properties of the atomic nucleus. Many scientific techniques exploit NMR phenomena to study molecular physics, crystals, and non-crystalline materials through NMR spectroscopy. NMR is also routinely used in advanced medical imaging techniques, such as in magnetic resonance imaging (MRI).
All isotopes that contain an odd number of protons and/or of neutrons (see Isotope) have an intrinsic magnetic moment and angular momentum, in other words a nonzero spin, while all nuclides with even numbers of both have a total spin of zero. The most commonly studied nuclei are 1H and 13C, although nuclei from isotopes of many other elements (e.g. 2H, 6Li, 10B, 11B, 14N, 15N, 17O, 19F, 23Na, 29Si, 31P, 35Cl, 113Cd, 129Xe, 195Pt) have been studied by high-field NMR spectroscopy as well.
A key feature of NMR is that the resonance frequency of a particular substance is directly proportional to the strength of the applied magnetic field. It is this feature that is exploited in imaging techniques; if a sample is placed in a non-uniform magnetic field then the resonance frequencies of the sample's nuclei depend on where in the field they are located. Since the resolution of the imaging technique depends on the magnitude of magnetic field gradient, many efforts are made to develop increased field strength, often usingsuperconductors. The effectiveness of NMR can also be improved using hyperpolarization, and/or using two-dimensional, three-dimensional and higher-dimensional multi-frequency techniques.
The principle of NMR usually involves two sequential steps:
- The alignment (polarization) of the magnetic nuclear spins in an applied, constant magnetic field H0.
- The perturbation of this alignment of the nuclear spins by employing an electro-magnetic, usually radio frequency (RF) pulse. The required perturbing frequency is dependent upon the static magnetic field (H0) and the nuclei of observation.
The two fields are usually chosen to be perpendicular to each other as this maximizes the NMR signal strength. The resulting response by the total magnetization (M) of the nuclear spins is the phenomenon that is exploited in NMR spectroscopy and magnetic resonance imaging. Both use intense applied magnetic fields (H0) in order to achieve dispersion and very high stability to deliver spectral resolution, the details of which are described by chemical shifts, the Zeeman effect, and Knight shifts (in metals).
NMR phenomena are also utilized in low-field NMR, NMR spectroscopy and MRI in the Earth's magnetic field (referred to as Earth's field NMR), and in several types ofmagnetometers.
Nuclear magnetic resonance (NMR) is a physical phenomenon in which nuclei in a magnetic field absorb and re-emit electromagnetic radiation. This energy is at a specific resonance frequency which depends on the strength of the magnetic field and the magnetic properties of the isotope of the atoms; in practical applications, the frequency is similar to VHF and UHF television broadcasts (60–1000 MHz). NMR allows the observation of specific quantum mechanical magnetic properties of the atomic nucleus. Many scientific techniques exploit NMR phenomena to study molecular physics, crystals, and non-crystalline materials through NMR spectroscopy. NMR is also routinely used in advanced medical imaging techniques, such as in magnetic resonance imaging (MRI).
Nuclear magnetic resonance was first described and measured in molecular beams by Isidor Rabi in 1938,[1] by extending the Stern–Gerlach experiment, and in 1944, Rabi was awarded the Nobel Prize in physics for this work.[2] In 1946, Felix Bloch and Edward Mills Purcell expanded the technique for use on liquids and solids, for which they shared theNobel Prize in Physics in 1952.[3][4]
Purcell had worked on the development of radar during World War II at the Massachusetts Institute of Technology's Radiation Laboratory. His work during that project on the production and detection of radio frequency power and on the absorption of such RF power by matter laid the foundation for Rabi's discovery of NMR.
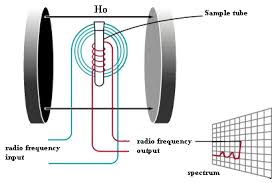
Rabi, Bloch, and Purcell observed that magnetic nuclei, like 1H and 31P, could absorb RF energy when placed in a magnetic field and when the RF was of a frequency specific to the identity of the nuclei. When this absorption occurs, the nucleus is described as being in resonance. Different atomic nuclei within a molecule resonate at different (radio) frequencies for the same magnetic field strength. The observation of such magnetic resonance frequencies of the nuclei present in a molecule allows any trained user to discover essential chemical and structural information about the molecule.
The development of NMR as a technique in analytical chemistry and biochemistry parallels the development of electromagnetic technology and advanced electronics and their introduction into civilian use.
Proton NMR
For a molecule such as diethyl ether, CH3CH2OCH2CH3, two types of protons would be predicted to appear in the NMR spectrum; a 'simple' CH3 in the area of
1, and a CH2 shifted down to about 4 by the electronegative oxygen. The NMR spectrum of diethyl ether, however, displays seven peaks, as shown below.
For a molecule such as diethyl ether, CH3CH2OCH2CH3, two types of protons would be predicted to appear in the NMR spectrum; a 'simple' CH3 in the area of
1, and a CH2 shifted down to about 4 by the electronegative oxygen. The NMR spectrum of diethyl ether, however, displays seven peaks, as shown below.
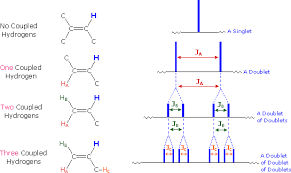
This multiplicity is due to the phenomena known as spin coupling and arises because of interaction of the proton magnetic field with bonding electrons. In essence, each proton can have one of two possible spin orientations in the applied field, so that the magnetic field sensed by adjacent protons can have one of two possible values. The result is that n protons will split adjacent protons into (n + 1) peaks. The intensities of these peaks are simply a result of the possible spin orientations, thus, the protons of a CH2 group can have the following possible spins:
The middle pair are degenerate, therefore a proton adjacent to a CH2 group will be split into three separate peaks in the ratio 1:2:1.
The separation between these peaks is referred to as the coupling constant, J which is measured in Hz; typical values for J seldom exceed 20 Hz and it is important to note that sets of coupled protons will display exactly the same coupling constant.
In the spectrum for diethyl ether, the CH3 group is split by the two protons on the adjacent CH2 group into three peaks (a triplet) and the absorbance for the CH2 is split by the three protons on the methyl group into (n + 1) = 4 peaks (a quartet). The two ethyl groups in diethyl ether show as a single absorbance since the molecule has a plane of symmetry, that is, both ethyl groups are chemically and magnetically equivalent.
The NMR spectrum of methyl isopropyl ether (CH3-CH(CH3)2) would be predicted to have three types of protons; the methyl group bonded to the oxygen, shifted to 4, a methyne proton (the CH), also shifted to the same region, and the isopropyl methyl protons, in a fairly 'simple' environment at 1. The CH3 bonded to the oxygen should appear as a single peak (a singlet) since it is not immediately adjacent to any protons. The CH should be split into seven peaks since it is adjacent to six equivalent hydrogens (the two CH3 groups) and the peak for the two (magnetically equivalent) CH3 groups should be split into two peaks (a doublet) by the adjacent CH. These predictions are consistent with the spectrum for methyl isopropyl ether shown below:
4, a methyne proton (the CH), also shifted to the same region, and the isopropyl methyl protons, in a fairly 'simple' environment at 1. The CH3 bonded to the oxygen should appear as a single peak (a singlet) since it is not immediately adjacent to any protons. The CH should be split into seven peaks since it is adjacent to six equivalent hydrogens (the two CH3 groups) and the peak for the two (magnetically equivalent) CH3 groups should be split into two peaks (a doublet) by the adjacent CH. These predictions are consistent with the spectrum for methyl isopropyl ether shown below:
The effects of splitting are often described using a splitting tree which depicts the original absorbance being split by a coupling constant J into (n + 1) peaks.
A splitting tree such as this is useful in understanding more complex splitting patterns, such as those that occur in Br-CH2CH2CH2-OD, as shown below:
As expected, the deuterium (2H) with spin = 1, does not show in the proton NMR. The methylene group adjacent to the bromine is shifted to 3.4 and the methylene adjacent to the oxygen is shifted to 3.75; both of these appear as simple triplets, each split by the central CH2. The coupling constants for these splitting patterns are slightly different; the ab constant is J = 15 Hz and the bc constant is J = 12 Hz (there is no simple way to predict this ahead of time). The splitting pattern for the central methylene is more complex, being split by the protons on carbon a into a triplet, J = 15 Hz, and each of these peaks being further split by the protons on carbonc into triplets with J = 12 Hz.
3.4 and the methylene adjacent to the oxygen is shifted to 3.75; both of these appear as simple triplets, each split by the central CH2. The coupling constants for these splitting patterns are slightly different; the ab constant is J = 15 Hz and the bc constant is J = 12 Hz (there is no simple way to predict this ahead of time). The splitting pattern for the central methylene is more complex, being split by the protons on carbon a into a triplet, J = 15 Hz, and each of these peaks being further split by the protons on carbonc into triplets with J = 12 Hz.
As shown by the splitting tree, the spectrum is predicted to consist of 9 peaks centered around 1.53. The actual NMR, as shown above, shows only five peaks in this region, This is because the central seven peaks are only separated by 2 Hz, and the 60 MHz spectrometer cannot resolve the individual peaks. Higher resolution spectrometers (i.e., 600 MHz) are useful at resolving fine structure in the spectrum such as this.
1.53. The actual NMR, as shown above, shows only five peaks in this region, This is because the central seven peaks are only separated by 2 Hz, and the 60 MHz spectrometer cannot resolve the individual peaks. Higher resolution spectrometers (i.e., 600 MHz) are useful at resolving fine structure in the spectrum such as this.
NMR is an abbreviation of Nuclear Magnetic Resonance. It involes with 3 parts;
Nuclear => nucleus (including proton & neutron)
Magnetic => external magnetic field
Resonance => resonance effect
(www.varianinc.com)
NMR spectroscopy is an absorption process that involes spinning of nuclei in an external magnetic field. It has many advantages such as;
allocating particular atoms in a molecule structural indentification measuring ratio of components in a mixture defining relative configuration 
All nuclei cary a positive charge, but only some have a charge spin on the nuclear axis which causes a magnetic dipole along the axis, therefore, posses an angular momentum. The angular momentum of spinning charge is described in term of spin quantum number (I), and then nuclei behave themselves like magnetic bars (only I
0).
When the external magnetic field is applied, nuclear magnets can orient themselves in 2I + 1 spin states (equal to the number of magnetic quantum number; m)
| where : | m | = magnetic quantum number |
| = +I, +I - 1, +I - 2, ..., -I + 2, -I + 1, -I |
The relationship of nuclei and magnetic propeties
nuclei
|
example
|
spin quantum no. (I)
|
magnetic quantum no. (m)
|
allowed spin states
|
activity in NMR
|
|
atomic mass
|
atomic no.
|
|||||
odd
|
odd or even
|
1H, 13C,19F, 31P
|
1/2
|
+1/2, -1/2
|
2(1/2) + 1 = 2
|
NMR active
|
35Cl
|
3/2
|
+3/2, 1/2, 0, -1/2, -3/2
|
2(3/2) + 1 = 4
|
NMR active
|
||
even
|
odd
|
2H, 14N
|
1
|
+1, 0, -1
|
2(1) + 1 = 3
|
NMR active
|
even
|
even
|
12C, 16O, 32S
|
0
|
0
|
2(0) + 1 = 1
|
NMR inactive
|
| No external magnetic field | |
The magnetic moments of protons are randomly oriented. |
| External magnetic field (B0) is applied | |
The protons orient themselves. Some (spin +1/2) are parallel (aligned) and some (spin -1/2) are antiparallel (against) to the applied magnetic field B0. |
For 1H and 13C nuclei, when the external magnetic field B0 is applied, only 2 orientations are allowed.
nuclei with spin +1/2 (a state) are parallel to B0 nuclei with spin -1/2 (b state) are antiparallel to B0
The energy difference between the allowed nuclear spin states for 1H nuclei
depends on the strength of the external magnetic field, B0 according to the equation :
| where | g = gyromagnetic ratio h = Planck's constant  = h/2p |
For a proton,
if the applied magnetic field has a strength of apploxymately 1.41 T,
the difference in energy between the 2 spin states is 2.39 x 10 kJ/mol.
Radiation with a frequency of about 60 MHz (RF region) corresponds to
this energy difference (See the table below).
Frequencies and field strengths at which selected nuclei have their nuclear resonances
isotope
|
natural abundance (%)
|
strength of B0 (Tesla, T)
|
energy difference between the two spin states (MHz)
|
1H
|
99.98
|
0
|
0
|
1.00
|
42.6
|
||
1.41
|
60
|
||
7.04
|
300
|
||
2H
|
0.0156
|
1.00
|
6.5
|
13C
|
1.108
|
1.00
|
10.7
|
1.41
|
15.1
|
||
7.04
|
75.0
|
||
19F
|
100.0
|
1.00
|
40.0
|
31P
|
100.0
|
1.00
|
17.2
|
We have known that ;
 |
If no external magnetic field (B0 = 0), the magnetic moments of protons are randomly oriented. |
| |
When an external magnetic field (B0) is applied in z direction, the protons orient themselves and precess with their precession frequencies. Some (spin +1/2) are parallel (aligned) and some (spin -1/2) are antiparallel (against) to the applied magnetic field B0. |
| |
If RF at the precession frequency is applied in x direction, the two frequencies (precession & Radio frequencies) couple, energy is absorbed, and then the nuclear spin flips from spin state +1/2 to spin state –1/2. This is called magnetic resonance. |
| |
The absorbed energy is detected in y direction, and then is transformed to a signal. |
protons (1H)
|
environment of 1H
|
absorbed RF
|
frequency
|
isolated 1H
|
same environment
|
all 1Hs absorb at the same RF and produce only one signal for all 1Hs
|
constant frequency
(indistinguishable) |
1H in a molecule
|
different environments
|
each 1H absorbs at different RF and produces signal for each different 1Hs
|
variable frequency
(distinguishable) |
How do nuclei in the higher energy state return to the lower state?
There are two major relaxation processes;
1. Non-radiation process => Spin - lattice (longitudinal) relaxation (T1)
2. Radiation process => Spin - spin (transverse) relaxation (T2)
We will focus on non-radiative relaxation processes (thermodynamics).
The sample in which the nuclei are held is called the lattice. Nuclei
in the lattice are in vibrational and rotational motion, which creates a
complex magnetic field, called lattice field.
This lattice field has many components that can interact with nuclei in
the higher energy state and cause them to lose energy and return to the
lower state. The lost energy increases the amount of vibration and
rotation within the lattice (resulting in a tiny rise in the temperature
of the sample).
The relaxation time, T1 (the average lifetime of nuclei in the higher energy state) depends on the gyromagnetic ratio (g) of the nucleus and the mobility of the lattice.
| |
As mobility increases, the vibrational and rotational frequencies increase, making it more likely for a component of the lattice field to be able to interact with excited nuclei. |
| |
At extremely high mobilities, the probability of a component of the lattice field being able to interact with excited nuclei decreases. |
Spin-spin relaxation or transverse relaxation (T2) describes the interaction between neighbouring nuclei with identical precession frequencies but differ in magnetic quantum states. In this situation, the nuclei can exchange quantum states; a nucleus in the lower energy level will be excited, while the excited nucleus relaxes to the lower energy state. There is no net change in the populations of the energy states, but the average lifetime of a nucleus in the excited state will decrease.
Long T2 relaxation times only occur in molecules which are tumbling rapidly in solution. Less movement nuclei and solids have rapid spin-spin relaxation.
In an NMR spectrometer, there are 4 important components ;
1. NMR Powerful Magnet
2. NMR Probe
3. NMR Console
radio-frequency generator
radio-frequency detector
signal amplifier
radio-frequency generator radio-frequency detector signal amplifier
4. Computer Workstation and
Control Software(or Data Processing)
Control Software(or Data Processing)
(Picture from www.varianinc.com)
At the beginning, an NMR spectrometer uses sweep mode of magnetic field.
Process of measurement:
1. The various or swept magnetic field strength is generated by a powerful megnet (z direction).
2. A sample is irradiated with radio frequency (RF) of a constant frequency (x direction).
3. When the magnetic field reaches the correct strength, the nuclei absorb energy and resonance occurs.
4. This absorption causes a tiny electrical current to flow in a receiver coil surrounding the sample (y direction).
5. The instrument then amplifies this current and displays it as a signal (peaks).
6. The signal recorded is NMR spectrum .
2. A sample is irradiated with radio frequency (RF) of a constant frequency (x direction).
3. When the magnetic field reaches the correct strength, the nuclei absorb energy and resonance occurs.
4. This absorption causes a tiny electrical current to flow in a receiver coil surrounding the sample (y direction).
5. The instrument then amplifies this current and displays it as a signal (peaks).
6. The signal recorded is NMR spectrum .
Diagram of a Continuous-Wave NMR Spectrometer
In
modern NMR spectrometers, the instrument uses the pulse of radio
frequency. The RF generator is turned on and off very quickly,
generating a pulse similar to that shown in Figure a). The pulse
contains a range of frequencies centered about the fundamental as shown
in Figure b).
Process of measurement:
1. The magnetic field is held constant.
2. The sample is irradiated with a short pulse (approximately 10-5 s) of radio-frequency energy.
3. The radio-frequency energy flips the spins of all susceptible nuclei simultaneously.
4. When the pulse is discontinued, the excited nuclei begin to lose their excitation energy and return to their original spin state, or relax. Since the molecule contains many diffferent nuclei, many diffferent frequencies of EM radiation are emitted simultaneously. This emission is called free-induction decay or FID signal as shown below.
2. The sample is irradiated with a short pulse (approximately 10-5 s) of radio-frequency energy.
3. The radio-frequency energy flips the spins of all susceptible nuclei simultaneously.
4. When the pulse is discontinued, the excited nuclei begin to lose their excitation energy and return to their original spin state, or relax. Since the molecule contains many diffferent nuclei, many diffferent frequencies of EM radiation are emitted simultaneously. This emission is called free-induction decay or FID signal as shown below.
5. The intensity of the FID decays with time and falls to zero as nuclei return to their equilibrium state.
If the signal did not decay in intensity, it would appear as a sine (or cosine) wave of constant intensity as shown below.
6. A computer records the intensity-versus-time information (time domain) and then uses a Fourier Transform to convert the information to intensity-versus-frequency information (frequency domain).
Diagram of an FTNMR spectrometer
The particular advantage of FTNMR spectrometer Low sample is required. 5 - 10 mins are required to scan and record a CW spectrum; a pulse experiment is much faster, a few seconds. A large number of spectra can be recorded and digitally summed to give a time-averaged spectrum. Instrumental electronic noise is random and partially cancelled out when spectra are time-averaged. Sample signals is accumulated and become much stronger relative to the noise than those from a single spectrum.
The signals in different magnetic fields show different positions in Hz. The term "chemical shift" is introduced to eliminate the changing position due to the shift in the strength of magnetic field.Consider the table below;
compound
|
strength of field; B0 (Tesla)
|
operating frequency (MHz)
|
position of signal (Hz)
|
chemical shift (ppm)
|
CH3-Br
|
1.41
|
60
|
162
|
2.70
|
2.35
|
100
|
270
|
2.70
|
Chemical shift (d) is the shift in part per million of an NMR signal from the signal of TMS.
If signal is shifted toward the left on the chart paper, it is shifted downfield (low magnetic field). If signal is shifted toward the right on the chart paper, it is shifted upfield (high magnetic field).
 |
The reference compound for 1H-NMR and 13C-NMR spectroscopy is tetramethylsilane (TMS) which the signal is assigned to be d
of 0 ppm. TMS is chosen initially because protons of its methyl groups
are more deshielded than those of other known compounds. At that time,
no compounds which had better shielded hydrogen than TMS were known. |
1H NMR spectrum of 1,4-dimethylbenzene
In a given molecule, some hydrogens posses more electron density and
some posses less electron density. Therefore, they absorb energy at
different frequencies and then they are recorded at different positions.
chemical shift (ppm)
|
signal from
|
7.2
|
Hb
|
2.3
|
Ha
|
The field strength (at which absorption occurs) is highly dependent on
the magnetic environment of each proton. The magnetic environment
depends on; the magnetic fields generated by its own electrons (circulating electrons) the magnetic fields resulted from other nearby protons (or other nuclei)
The
relative magnitudes of the signals are helpful in assignment the number
of protons in each group. The areas of the signal are plotted and
recorded as an integral curve.
Example : From the integration, calculate the number of hydrogens giving rise to each carbon.
The total integration is 88 mm and corresponds to 10 hydrogens.
chemical shift (ppm)
|
calculation
|
no. of hydrogens
|
7.24
|
(44/88) x 10
|
5
|
5.10
|
(18/88) x 10
|
2
|
2.14
|
(26/88) x 10
|
3
|
When one nucleus is influenced by another nucleus with spin quantum number I0, the two nuclei are said to be coupled, both will show 2 lines instead of 1 line for each nucleus.
The reason is that the field caused by Hx can be parallel or antiparallel so the Ha can experience not only the applied field but also combination of both fields. One signal absorbs at higher field (if Hx is parallel) and one signal absorbs at lower field (if Hx is antiparallel). The two lines are observed for each nucleus. This is called spin-spin splitting. The signal of Ha is split into 2 peaks (doublet) because there is spin-spin coupling between nonequivalent protons (Ha and Hx).
In order to obtain the signals, the B0 will be adjusted to maintain the resonance conditions, either lower or higher field than without Hx. Each peak of the doublet has nearly equal area and is half in the absence of Hx because the population of Ha in the two spin states are nearly equal according to the Boltzmann Distribution.- Since Ha and Hx is split by each other, the distance between 2 lines of both Ha and Hx signals is equal.
- If the two nuclei are only neighbors, then their signals are 2 lines (doublet).
The number of line splitting can be depicted by using the following equation;
multiplicity = 2nI + 1, where n is no. of neighboring protons.
nuclei
|
I
|
multiplicity
|
1H
|
1/2
|
n + 1
|
2H and 14N
|
1
|
2n + 1
|
35Cl
|
3/2
|
3n + 1
|
Since the spin quantum no. (I) of 1H is 1/2, therefore,
multiplicity = 2n + 1
Consider these examples,
Splitting patterns for a proton with 0, 1, 2, and 3 equivalent neighboring protons
Peak areas in any multiplet can be derived from Pascal's triangle
The signal splitting in 1H-NMR spectra is usually small, ranging from fractions of a Hz to as much as 18 Hz, and is designated as J (referred to the coupling constant).
Coupling Constants (J)
J is the distance between peaks in a splitting signal
the magnitude of J is
- dependent only on fields that are caused by magnetic atoms within a molecule but
- independent of the external field strength (B0)
J is the distance between peaks in a splitting signal the magnitude of J is - dependent only on fields that are caused by magnetic atoms within a molecule but
- independent of the external field strength (B0)
The most common patterns that have been seen are doublet and triplet.
 |
 |
n = 1
|
n = 2
|
Spectra that can be interpreted by using n+1 rule are said to be first-order spectra. In first-order spectra, the difference in chemical shifts (in Hz) between 2 groups of protons is large. When Dn/J is large and we see first-order splitting, the system is said to be weakly coupled.
In some spectra, using the n+1 rule is not enough, therefore, such advanced analysis may be required for interpreting spectra. These spectra are said to be second-order spectra. In second-order spectra, the difference in chemical shifts between 2 groups of protons is similar in magnitude to the J. When Dn/J is large and we see second-order splitting, the system is said to be strongly coupled. 
1. The ethyl acetate spectrum displays the typical quartet and triplet of a substituted ethyl group.
Example of second-order spetra
If a given nucleus is spin-coupled to two or more sets of neighboring nuclei by different J values, the n+1 rule does not predict the entire splitting pattern. The spectra may be complicated due to the strong coupling.
These are some examples of more complicated spectra that can be 2nd order spectra.
Magnitude of Some Typical Coupling Constants
Spin - Spin Coupling => the way in which the spin of one nucleus influences spin of another
Dirac vector model The
electrons in the intervening bonds between the two nuclei transfer spin
information from one to another by means of interation between the
nuclear and electronic spin.
An
electron near the nucleus is assumed to have the lowest energy of
interation with the the nucleus when the spin of the electron has its
spin direction paired to that of the nucleus.Types of Spin-Spin Coupling
Dirac model makes it easy to understand why the size of J decreases as the no. of intervening bonds increases.
2. Two-Bond Coupling (2J) => Geminal Coupling
3. Three-Bond Coupling (3J) => Vicinal Coupling
3. Long Range Coupling (4J, 5J, ...)
1H NMR OF SIMPLE MOLECULES.. Brush up
ما هو هذا، لماذا أنت قلق، فرشاة مع الأشياء البسيطة، وسوف يخرج عبقري، وتعلم معي، قطرات صغيرة من الماء جعلالمحيطات، وسوف يكون خبيرا في هذا
what is this, why are you worried, brush up with simple things, you will come out genius, learn with me, small drops of water make an ocean, you will be an expert in this
あなたが心配している理由は、これは簡単なことでブラッシュアップ、、何か、あなたは、天才が出てくる私と一緒に学ぶことが、水の小さな滴が海を作るには、この専門家になる
4-methylbenzaldehyde
The proton NMR has three peaks; a singlet at 2.2 (3H), and a singlet at 10 (1H) and two doublets centered around 7.6. The doublets centered at 7.6 are in the aromatic region; the fact that two doublets are observed (2H each) suggests a 1,4-disubstituted aromatic compound.
The peak at 2.2 is in the region for a methyl group adjacent a mildly electronegative group. The singlet at 10 is in the region observed for aldehydic protons. The presence of two doublets in the aromatic region is highly characteristic of 1,4-disubstitution.
τι είναι αυτό, γιατί είσαι ανήσυχος, βούρτσα με απλά πράγματα, θα βγει ιδιοφυΐα, να μάθουν μαζί μου, μικρές σταγόνες του νερού κάνει έναν ωκεανό, θα είστε ένας εμπειρογνώμονας σε αυτό
methyl acetate (methyl ethanoate)
The proton NMR has two singlets of equal area. The fact that the molecule has 6 hydrogens means that there must be two non-identical CH3 groups in the molecule and that they are non-adjacent. The peak at 1.95 is in the area generally observed for methyl groups adjacent to carbonyls; the peak at 3.85 is in the region for methyl groups adjacent to electronegative atoms, i.e., oxygen.
আপনি চিন্তিত কেন এই সহজ জিনিস নিয়ে ব্রাশ,, কি, আপনি, প্রতিভা বাইরে আসতে আমার সাথে শিখতে হবে, জলের ছোট ঝরিয়া একটি মহাসাগর না, আপনি এই একজন বিশেষজ্ঞ হতে হবে
The proton NMR has a quartet and a triplet, indicating a CH2 adjacent to a CH3. The peak at 2.5 is in the area generally observed for methyl groups adjacent to mildly electronegative groups; the peak at 1.2 is in the region for simple methyl groups adjacent to carbons (CH3CH2). The molecule contains oxygen, but the peak at 2.5 is not shifted enough for this to represent an -O- linkage, so it must represent a carbonyl.
מה זה, למה אתה מודאג, לרענן עם דברים פשוטים, אתה תצא גאון, ללמוד איתי, טיפות קטנות של מיםיגרמו לים, אתה תהיה מומחה בזה
acetaldehyde (ethanal)
The proton NMR has two peaks; at high resolution, fine coupling is evident between them (J 1 Hz) with the peak at 9.7 being a quartet and the peak at 2.1 being a doublet, indicating a CH coupled to a CH3. The peak at 9.7 is in the area generally observed for aldehydic protons; the peak at 2.1 is in the region for a methyl group adjacent a carbonyl. The molecule contains oxygen, but the peak at 2.1 is not shifted enough for this to represent an -O- linkage, so it must represent a carbonyl. The very small coupling constant suggests that the proton and the CH3 group are not immediately adjacent, but that this represents an example of cross-space coupling.
o que é isso, por que você está preocupado, retocar com coisas simples, você vai sair gênio, aprender comigo, pequenas gotas de água fazem um oceano, você vai ser um especialista neste
Name: ethyl acetate (ethyl ethanoate)
The proton NMR has three peaks; a quartet at 4.1 (2H), a triplet at 1.2 (3H) and a singlet at 1.97 (3H). The quartet and triplet suggest a CH2 coupled to a CH3 in an ethyl group. The peak at 4.1 is in the area generally observed for CH groups adjacent to electronegative groups, i.e., oxygen. The peak at 2 is in the region for a methyl group adjacent a carbonyl.
நீங்கள் கவலை ஏன் இந்த எளிய பொருட்களை கொண்டு துலக்க, என்ன, நீங்கள், மேதை வெளியே வர எனக்கு கற்று, நீர் சிறு துளிகள் ஒரு கடல் செய்ய, நீங்கள் இந்த ஒரு நிபுணர் இருக்கும்
के तपाईं चिंतित हो किन यो सरल कुरा संग ब्रश,, के हो, तपाईं, प्रतिभा बाहिर आउन मलाई संग सिक्न हुनेछ, पानी सानो थोपा एक महासागर बनाउन, तपाईं यस मा एक विशेषज्ञ हुनेछ\
તમને ચિંતા થતી હોય કે શા માટે આ સરળ બાબતો સાથે બ્રશ, શું છે, તમે પ્રતિભા બહાર આવે મારી સાથે શીખશે, પાણી નાના ટીપાં સમુદ્ર કરો, તો તમે આ એક નિષ્ણાત હશે
kas tas ir, kāpēc jūs uztraucaties, suka ar vienkāršām lietām, jūs iznākt ģēnijs, mācīties kopā ar mani, nelieli ūdens pilieni veikt okeānu, jums būs eksperts šajā
что это такое, почему ты беспокоишься, освежить с простых вещей, вы будете выходить гений, узнать со мной, маленькие капли воды сделать океан, вы будете экспертом в этом
hvað er þetta, af hverju ert þú áhyggjur, bursta upp með einföldum hlutum, verður þú að koma út snillingur, læra með mér, litla dropa af vatni gera haf, verður þú að vera sérfræðingur í þessu
The proton NMR has four groups of peaks; the singlet at 3.6 (3H) is consistent with an isolated CH3 group adjacent to an electronegative center, such as oxygen. The sets of highly split doublets centered at 6.5, 4.2 and 4.0 (there is overlap of these at 4.1) span the region where alkene hydrogens are observed. The complex splitting pattern observed resembles an ABC pattern for a terminal alkene.
这是什么，你为什么担心，刷了简单的事情，你会出来的天才，学我，小水珠做出的海洋，你将在这方面的专家
당신이 걱정하는 이유는 간단한 것들로 브러시, 무엇인가, 당신은 천재 나올 나와 함께 배울 것, 물 작은 방울은 바다를 만들어,이 분야의 전문가가 될 것입니다
The proton NMR has two peaks; both singlets are consistent with isolated groups adjacent to an electronegative center, such as oxygen. The fact that the molecule contains ten hydrogens, but only shows singlets for two and three hydrogens requires a great deal of symmetry in the molecule, i.e., both CH3 groups must be identical, as must both CH2 groups.
The proton NMR has four sets of peaks; a singlet at 3.6 (3H), two sets of doublets centered around 6.9 (4H), a septet at 2.7 (1H) and a doublet at 1.6. The singlet at 3.6 is consistent with an isolated CH3adjacent to an electronegative center, such as an oxygen. The septet and doublet strongly suggest an isopropyl group 
CH(CH3) 2 in which the carbon is bonded to something mildly electronegative and the two doublets centered at 6.9 strongly suggest a 1,4-disubstituted aromatic compound.
The proton NMR has three sets of peaks; a singlet at 4.3 (2H), a messy singlet around 7.1 (4H), and a singlet at 2.3 (3H). The singlet at 2.3 is consistent with an isolated CH3 adjacent to a mildly electronegative center. The singlet at 4.3 is consistent with a CH2 group adjacent to two mildly electronegative centers (X-CH2-Y), and the singlet at 7.1 is consistent with a disubstituted aromatic compound. The substitution pattern is not what is normally seen in 1,4-disubstitution (two doublets), suggesting 1,2 or 1,3-disubstitution.
آپ پریشان کیوں ہیں اس سادہ چیزوں کے ساتھ برش،، کیا ہے، آپ، ہوشیار باہر آ میرے ساتھ سیکھ جائے گی، پانی کے چھوٹے چھوٹے قطرے ایک سمندر بنانے کے لئے، آپ کو اس میں ایک ماہر ہو جائے گا
THANKS AND REGARD’S
DR ANTHONY MELVIN CRASTO Ph.D
MOBILE-+91 9323115463
GLENMARK SCIENTIST , INDIA
web link
http://anthonycrasto.jimdo.com/
Congratulations! Your presentation titled “Anthony Crasto Glenmark scientist, helping millions with websites” has just crossed MILLION views.
アンソニー 安东尼 Энтони 안토니 أنتوني
join my process development group on google
you can post articles and will be administered by me on the google group which is very popular across the world
LinkedIn group
blogs are 
ما هو هذا، لماذا أنت قلق، فرشاة مع الأشياء البسيطة، وسوف يخرج عبقري، وتعلم معي، قطرات صغيرة من الماء جعلالمحيطات، وسوف يكون خبيرا في هذا
what is this, why are you worried, brush up with simple things, you will come out genius, learn with me, small drops of water make an ocean, you will be an expert in this
あなたが心配している理由は、これは簡単なことでブラッシュアップ、、何か、あなたは、天才が出てくる私と一緒に学ぶことが、水の小さな滴が海を作るには、この専門家になる
4-methylbenzaldehyde
The proton NMR has three peaks; a singlet at 2.2 (3H), and a singlet at 10 (1H) and two doublets centered around 7.6. The doublets centered at 7.6 are in the aromatic region; the fact that two doublets are observed (2H each) suggests a 1,4-disubstituted aromatic compound.
2.2 (3H), and a singlet at 10 (1H) and two doublets centered around 7.6. The doublets centered at 7.6 are in the aromatic region; the fact that two doublets are observed (2H each) suggests a 1,4-disubstituted aromatic compound.
The peak at 2.2 is in the region for a methyl group adjacent a mildly electronegative group. The singlet at 10 is in the region observed for aldehydic protons. The presence of two doublets in the aromatic region is highly characteristic of 1,4-disubstitution.
2.2 is in the region for a methyl group adjacent a mildly electronegative group. The singlet at 10 is in the region observed for aldehydic protons. The presence of two doublets in the aromatic region is highly characteristic of 1,4-disubstitution.
τι είναι αυτό, γιατί είσαι ανήσυχος, βούρτσα με απλά πράγματα, θα βγει ιδιοφυΐα, να μάθουν μαζί μου, μικρές σταγόνες του νερού κάνει έναν ωκεανό, θα είστε ένας εμπειρογνώμονας σε αυτό
methyl acetate (methyl ethanoate)
The proton NMR has two singlets of equal area. The fact that the molecule has 6 hydrogens means that there must be two non-identical CH3 groups in the molecule and that they are non-adjacent. The peak at 1.95 is in the area generally observed for methyl groups adjacent to carbonyls; the peak at 3.85 is in the region for methyl groups adjacent to electronegative atoms, i.e., oxygen.
1.95 is in the area generally observed for methyl groups adjacent to carbonyls; the peak at 3.85 is in the region for methyl groups adjacent to electronegative atoms, i.e., oxygen.
আপনি চিন্তিত কেন এই সহজ জিনিস নিয়ে ব্রাশ,, কি, আপনি, প্রতিভা বাইরে আসতে আমার সাথে শিখতে হবে, জলের ছোট ঝরিয়া একটি মহাসাগর না, আপনি এই একজন বিশেষজ্ঞ হতে হবে
The proton NMR has a quartet and a triplet, indicating a CH2 adjacent to a CH3. The peak at 2.5 is in the area generally observed for methyl groups adjacent to mildly electronegative groups; the peak at 1.2 is in the region for simple methyl groups adjacent to carbons (CH3CH2). The molecule contains oxygen, but the peak at 2.5 is not shifted enough for this to represent an -O- linkage, so it must represent a carbonyl.
2.5 is in the area generally observed for methyl groups adjacent to mildly electronegative groups; the peak at 1.2 is in the region for simple methyl groups adjacent to carbons (CH3CH2). The molecule contains oxygen, but the peak at 2.5 is not shifted enough for this to represent an -O- linkage, so it must represent a carbonyl.
מה זה, למה אתה מודאג, לרענן עם דברים פשוטים, אתה תצא גאון, ללמוד איתי, טיפות קטנות של מיםיגרמו לים, אתה תהיה מומחה בזה
acetaldehyde (ethanal)
The proton NMR has two peaks; at high resolution, fine coupling is evident between them (J 1 Hz) with the peak at 9.7 being a quartet and the peak at 2.1 being a doublet, indicating a CH coupled to a CH3. The peak at 9.7 is in the area generally observed for aldehydic protons; the peak at 2.1 is in the region for a methyl group adjacent a carbonyl. The molecule contains oxygen, but the peak at 2.1 is not shifted enough for this to represent an -O- linkage, so it must represent a carbonyl. The very small coupling constant suggests that the proton and the CH3 group are not immediately adjacent, but that this represents an example of cross-space coupling.
1 Hz) with the peak at 9.7 being a quartet and the peak at 2.1 being a doublet, indicating a CH coupled to a CH3. The peak at 9.7 is in the area generally observed for aldehydic protons; the peak at 2.1 is in the region for a methyl group adjacent a carbonyl. The molecule contains oxygen, but the peak at 2.1 is not shifted enough for this to represent an -O- linkage, so it must represent a carbonyl. The very small coupling constant suggests that the proton and the CH3 group are not immediately adjacent, but that this represents an example of cross-space coupling.
o que é isso, por que você está preocupado, retocar com coisas simples, você vai sair gênio, aprender comigo, pequenas gotas de água fazem um oceano, você vai ser um especialista neste
Name: ethyl acetate (ethyl ethanoate)
The proton NMR has three peaks; a quartet at 4.1 (2H), a triplet at 1.2 (3H) and a singlet at 1.97 (3H). The quartet and triplet suggest a CH2 coupled to a CH3 in an ethyl group. The peak at 4.1 is in the area generally observed for CH groups adjacent to electronegative groups, i.e., oxygen. The peak at 2 is in the region for a methyl group adjacent a carbonyl.
4.1 (2H), a triplet at 1.2 (3H) and a singlet at 1.97 (3H). The quartet and triplet suggest a CH2 coupled to a CH3 in an ethyl group. The peak at 4.1 is in the area generally observed for CH groups adjacent to electronegative groups, i.e., oxygen. The peak at 2 is in the region for a methyl group adjacent a carbonyl.
நீங்கள் கவலை ஏன் இந்த எளிய பொருட்களை கொண்டு துலக்க, என்ன, நீங்கள், மேதை வெளியே வர எனக்கு கற்று, நீர் சிறு துளிகள் ஒரு கடல் செய்ய, நீங்கள் இந்த ஒரு நிபுணர் இருக்கும்
के तपाईं चिंतित हो किन यो सरल कुरा संग ब्रश,, के हो, तपाईं, प्रतिभा बाहिर आउन मलाई संग सिक्न हुनेछ, पानी सानो थोपा एक महासागर बनाउन, तपाईं यस मा एक विशेषज्ञ हुनेछ\
તમને ચિંતા થતી હોય કે શા માટે આ સરળ બાબતો સાથે બ્રશ, શું છે, તમે પ્રતિભા બહાર આવે મારી સાથે શીખશે, પાણી નાના ટીપાં સમુદ્ર કરો, તો તમે આ એક નિષ્ણાત હશે
kas tas ir, kāpēc jūs uztraucaties, suka ar vienkāršām lietām, jūs iznākt ģēnijs, mācīties kopā ar mani, nelieli ūdens pilieni veikt okeānu, jums būs eksperts šajā
что это такое, почему ты беспокоишься, освежить с простых вещей, вы будете выходить гений, узнать со мной, маленькие капли воды сделать океан, вы будете экспертом в этом
hvað er þetta, af hverju ert þú áhyggjur, bursta upp með einföldum hlutum, verður þú að koma út snillingur, læra með mér, litla dropa af vatni gera haf, verður þú að vera sérfræðingur í þessu
methyl vinyl ether (methyl ethenyl ether)
The proton NMR has four groups of peaks; the singlet at 3.6 (3H) is consistent with an isolated CH3 group adjacent to an electronegative center, such as oxygen. The sets of highly split doublets centered at 6.5, 4.2 and 4.0 (there is overlap of these at 4.1) span the region where alkene hydrogens are observed. The complex splitting pattern observed resembles an ABC pattern for a terminal alkene.
3.6 (3H) is consistent with an isolated CH3 group adjacent to an electronegative center, such as oxygen. The sets of highly split doublets centered at 6.5, 4.2 and 4.0 (there is overlap of these at 4.1) span the region where alkene hydrogens are observed. The complex splitting pattern observed resembles an ABC pattern for a terminal alkene.
这是什么，你为什么担心，刷了简单的事情，你会出来的天才，学我，小水珠做出的海洋，你将在这方面的专家
당신이 걱정하는 이유는 간단한 것들로 브러시, 무엇인가, 당신은 천재 나올 나와 함께 배울 것, 물 작은 방울은 바다를 만들어,이 분야의 전문가가 될 것입니다
Name: 1,2-dimethoxyethane
The proton NMR has two peaks; both singlets are consistent with isolated groups adjacent to an electronegative center, such as oxygen. The fact that the molecule contains ten hydrogens, but only shows singlets for two and three hydrogens requires a great deal of symmetry in the molecule, i.e., both CH3 groups must be identical, as must both CH2 groups.
4-isopropyl-1-methoxybenzene (4-(1-methylethyl)-1-methoxybenzene)
The proton NMR has four sets of peaks; a singlet at 3.6 (3H), two sets of doublets centered around 6.9 (4H), a septet at 2.7 (1H) and a doublet at 1.6. The singlet at 3.6 is consistent with an isolated CH3adjacent to an electronegative center, such as an oxygen. The septet and doublet strongly suggest an isopropyl group CH(CH3) 2 in which the carbon is bonded to something mildly electronegative and the two doublets centered at 6.9 strongly suggest a 1,4-disubstituted aromatic compound.
3.6 (3H), two sets of doublets centered around 6.9 (4H), a septet at 2.7 (1H) and a doublet at 1.6. The singlet at 3.6 is consistent with an isolated CH3adjacent to an electronegative center, such as an oxygen. The septet and doublet strongly suggest an isopropyl group CH(CH3) 2 in which the carbon is bonded to something mildly electronegative and the two doublets centered at 6.9 strongly suggest a 1,4-disubstituted aromatic compound.
3-bromomethyltoluene (3-bromomethyl-1-methylbenzene)
The proton NMR has three sets of peaks; a singlet at 4.3 (2H), a messy singlet around 7.1 (4H), and a singlet at 2.3 (3H). The singlet at 2.3 is consistent with an isolated CH3 adjacent to a mildly electronegative center. The singlet at 4.3 is consistent with a CH2 group adjacent to two mildly electronegative centers (X-CH2-Y), and the singlet at 7.1 is consistent with a disubstituted aromatic compound. The substitution pattern is not what is normally seen in 1,4-disubstitution (two doublets), suggesting 1,2 or 1,3-disubstitution.
4.3 (2H), a messy singlet around 7.1 (4H), and a singlet at 2.3 (3H). The singlet at 2.3 is consistent with an isolated CH3 adjacent to a mildly electronegative center. The singlet at 4.3 is consistent with a CH2 group adjacent to two mildly electronegative centers (X-CH2-Y), and the singlet at 7.1 is consistent with a disubstituted aromatic compound. The substitution pattern is not what is normally seen in 1,4-disubstitution (two doublets), suggesting 1,2 or 1,3-disubstitution.
آپ پریشان کیوں ہیں اس سادہ چیزوں کے ساتھ برش،، کیا ہے، آپ، ہوشیار باہر آ میرے ساتھ سیکھ جائے گی، پانی کے چھوٹے چھوٹے قطرے ایک سمندر بنانے کے لئے، آپ کو اس میں ایک ماہر ہو جائے گا
THANKS AND REGARD’S
DR ANTHONY MELVIN CRASTO Ph.D
DR ANTHONY MELVIN CRASTO Ph.D
MOBILE-+91 9323115463
GLENMARK SCIENTIST , INDIA
web link
web link
http://anthonycrasto.jimdo.com/
blogs are
Congratulations! Your presentation titled “Anthony Crasto Glenmark scientist, helping millions with websites” has just crossed MILLION views.
アンソニー 安东尼 Энтони 안토니 أنتوني
join my process development group on google
you can post articles and will be administered by me on the google group which is very popular across the world
LinkedIn group
blogs are
////////////////
This is the first time I am visiting your website. I would like to tell you that I am genuinely engaged by reading your blogs. Keep up the good work! injection bonding magnets manufacturer
ReplyDeleteGreat Blog, there is so much reality written in this content and everything is very hard to be argued. A top-notch blog having excellent content.injection bonding magnets supplier
ReplyDelete